Prompted, as described in the previous post, by Craig Callender's post on the uncertainty principle, I've gone back to David Bohm's original series of two papers "A suggested interpretation of the quantum theory in terms of "hidden" variables I" and "...II", published in Physical Review in 1952 (and reprinted in Wheeler and Zurek's classic collection "Quantum Theory and Measurement", Princeton University Press, 1983). The Bohm papers and others appear to be downloadable here.
Question 1 of my previous post asked whether it is true that
"a "measurement of position" does not measure the pre-existing value of the variable called, in the theory, "position". That is, if one considers a single trajectory in phase space (position and momentum, over time), entering an apparatus described as a "position measurement apparatus", that apparatus does not necessarily end up pointing to, approximately, the position of the particle when it entered the apparatus."
It is fairly clear from Bohm's papers that the answer is "Yes". In section 5 of the second paper, he writes
"in the measurement of an "observable," Q, we cannot obtain enough information to provide a complete specification of the state of an electron, because we cannot infer the precisely defined values of the particle momentum and position, which are, for example, needed if we wish to make precise predictions about the future behavior of the electron. [...] the measurement of an "observable" is not really a measurement of any physical property belonging to the observed system alone. Instead, the value of an "observable" measures only an incompletely predictable and controllable potentiality belonging just as much to the measuring apparatus as to the observed system itself."
Since the first sentence quoted says we cannot infer precise values of "momentum and position", it is possible to interpret it as referring to an uncertainty-principle-like tradeoff of precision in measurement of one versus the other, rather than a statement that it is not possible to measure either precisely, but I think that would be a misreading, as the rest of the quote, which clearly concerns any single observable, indicates. Later in the section, he unambiguously gives the answer "Yes" to a mutation of my Question 1 which substitutes momentum for position. Indeed, most of the section is concerned with using momentum measurement as an example of the general principle that the measurements described by standard quantum theory, when interpreted in his formalism, do not measure pre-existing properties of the measured system.
Here's a bit of one of two explicit examples he gives of momentum measurement:
"...consider a stationary state of an atom, of zero angular momentum. [...] the
-field for such a state is real, so that we obtain
Thus, the particle is at rest. Nevertheless, we see from (14) and (15) that if the momentum "observable" is measured, a large value of this "observable" may be obtained if the
-field happens to have a large fourier coefficient,
, for a high value of
. The reason is that in the process of interaction with the measuring apparatus, the
-field is altered in such a way that it can give the electron particle a correspondingly large momentum, thus transferring some of the potential energy of interaction of the particle with its
-field into kinetic energy."
Note that the Bohmian theory involves writing the complex-valued wavefunction 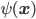 as
as 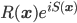 , i.e. in terms of its (real) modulus
, i.e. in terms of its (real) modulus 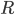 and (real) phase
and (real) phase  . Expressing the Schrödinger equation in terms of these variables is in fact probably what suggested the interpretation, since one gets something resembling classical equations of motion, but with a term that looks like a potential, but depends on
. Expressing the Schrödinger equation in terms of these variables is in fact probably what suggested the interpretation, since one gets something resembling classical equations of motion, but with a term that looks like a potential, but depends on 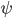 . Then one takes these classical-like equations of motion seriously, as governing the motions of actual particles that have definite positions and momenta. In order to stay in agreement with quantum theory concerning observed events such as the outcomes of measurements, m theory, one in addition keeps, from quantum theory, the assumption that the wavefunction
. Then one takes these classical-like equations of motion seriously, as governing the motions of actual particles that have definite positions and momenta. In order to stay in agreement with quantum theory concerning observed events such as the outcomes of measurements, m theory, one in addition keeps, from quantum theory, the assumption that the wavefunction 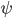 evolves according to the Schrödinger equation. And one assumes that we don't know the particles' exact position but only that this is distributed with probability measure given (as quantum theory would predict for the outcome of a position measurement) by
evolves according to the Schrödinger equation. And one assumes that we don't know the particles' exact position but only that this is distributed with probability measure given (as quantum theory would predict for the outcome of a position measurement) by 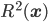 , and that the momentum is
, and that the momentum is 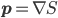 . That's why the real-valuedness of the wavefunction implies that momentum is zero: because the momentum, in Bohmian theory, is the gradient of the phase of the wavefunction.
. That's why the real-valuedness of the wavefunction implies that momentum is zero: because the momentum, in Bohmian theory, is the gradient of the phase of the wavefunction.
For completeness we should reproduce Bohm's (15).
(15) 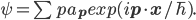
At least in the Wheeler and Zurek book, the equation has 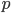 instead of
instead of 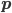 as the subscript on
as the subscript on 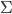 , and
, and 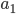 instead of
instead of 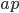 ; I consider these typos, and have corrected them. (Bohm's reference to (14), which is essentially the same as (15) seems to me to be redundant.)
; I consider these typos, and have corrected them. (Bohm's reference to (14), which is essentially the same as (15) seems to me to be redundant.)
The upshot is that
"the actual particle momentum existing before the measurement took place is quite different from the numerical value obtained for the momentum "observable,"which, in the usual interpretation, is called the "momentum." "
It would be nice to have this worked out for a position measurement example, as well. The nicest thing, from my point of view, would be an example trajectory, for a definite initial position, under a position-measurement interaction, leading to a final position different from the initial one. I doubt this would be too hard, although it is generally considered to be the case that solving the Bohmian equations of motion is difficult in the technical sense of complexity theory. I don't recall just how difficult, but more difficult than solving the Schrödinger equation, which is sometimes taken as an argument against the Bohmian interpretation: why should nature do all that work, only to reproduce, because of the constraints mentioned above---distribution of 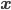 according to
according to 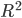 ,
, 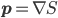 ---observable consequences that can be more easily calculated using the Schrödinger equation?
---observable consequences that can be more easily calculated using the Schrödinger equation?
I think I first heard of this complexity objection (which is of course something of a matter of taste in scientific theories, rather than a knockdown argument) from Daniel Gottesman, in a conversation at one of the Feynman Fests at the University of Maryland, although Antony Valentini (himself a Bohmian) has definitely stressed the ability of Bohmian mechanics to solve problems of high complexity, if one is allowed to violate the constraints that make it observationally indistinguishable from quantum theory. It is clear from rereading Bohm's 1952 papers that Bohm was excited about the physical possibility of going beyond these constraints, and thus beyond the limitations of standard quantum theory, if his theory was correct.
In fairness to Bohmianism, I should mention that in these papers Bohm suggests that the constraints that give standard quantum behavior may be an equilibrium, and in another paper he gives arguments in favor of this claim. Others have since taken up this line of argument and done more with it. I'm not familiar with the details. But the analogy with thermodynamics and statistical mechanics breaks down in at least one respect, that one can observe nonequilibrium phenomena, and processes of equilibration, with respect to standard thermodynamics, but nothing like this has so far been observed with respect to Bohmian quantum theory. (Of course that does not mean we shouldn't think harder, guided by Bohmian theory, about where such violations might be observed... I believe Valentini has suggested some possibilities in early-universe physics.)

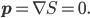
I think it is possible to do a position measurement in Bohmian mechanics in such a way that it simply reveals the pre-existing position of the particle. Simply start the measurement device in a position eigenstate, assume system and measuring device have no internal Hamiltonian and evolve under an interaction Hamiltonian that couples the position operator of the particle to the momentum operator of the measuring device. Of course, no realistic measuring device does this and even other idealized measuring procedures won't necessarily give the same result, but I think at least this works.
I think the comparison between nonequilibrium Bohmian mechanics and statistical mechanics is a bit unfair. In order to accept statistical mechanics, people did not demand that we see nonequilibrium phenomena. That came much later. What did convince people of statistical mechanics was the observation of small fluctuations away from equilibrium, e.g. Brownian motion. In the case of Bohmian mechanics, what we observe in quantum theory is analagous to equilibrium with fluctuations rather than to equilibrium itself because we observe individual measurement outcomes that only average to the Born rule probabilities over a long run of experiments. Therefore, it is a double standard to accept statistical mechanics on the basis of things like Brownian motion only whilst simultaneously arguing that Bohmian mechanics is implausible until we observe nonequilibrium phenomena.
Hi Matt---
Thanks for the insightful comments!
I agree that one can measure position, and reveal the existing value of the the particle's Bohmian position, if one starts with a position eigenstate. And similarly for momentum. I almost mentioned this in the post, but didn't want it to get too long. It is probably important enough that I should have done so, so thanks for bringing it up. [EDIT, AUGUST 26: Aha, your point is not the one I almost mentioned.....I misread your comment as assuming the system starts in a position eigenstate. So I will have to think about the implications of your comment some more...] The point I wanted to make, and that Bohm makes for the example of momentum measurements, is that this isn't what happens in general...if we don't start with a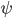 -field (Bohm's term for wavefunction), representing an eigenstate of momentum, then when we follow an individual trajectory of measured particle and measurement apparatus, in phase space, through a "momentum measurement procedure," we don't in general end up with the apparatus pointer pointing to the initial momentum of the measured particle. Of course Bohm postulates that in measurements of the sort we've so far been able to make, we don't directly see this sort of phenomenon because if the
-field (Bohm's term for wavefunction), representing an eigenstate of momentum, then when we follow an individual trajectory of measured particle and measurement apparatus, in phase space, through a "momentum measurement procedure," we don't in general end up with the apparatus pointer pointing to the initial momentum of the measured particle. Of course Bohm postulates that in measurements of the sort we've so far been able to make, we don't directly see this sort of phenomenon because if the 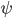 -field corresponds to a spread-out distribution over position and/or momentum, we can't know which trajectory we're on to begin with.
-field corresponds to a spread-out distribution over position and/or momentum, we can't know which trajectory we're on to begin with.
I'll have to think more about whether the arguments I gave about the analogy to statistical mechanics involve a double standard. That part of the post was a bit of an aside, and I didn't think it out very carefully. As far as "fluctuations" being behind the acceptance of a statistical mechanical account of thermodynamical phenomena, I agree that that is reasonable: it is basically a matter of, when you see probabilistic phenomena, asking whether there is some underlying reality such that the uncertainty in the phenomena can be ascribed to, and better understood, in terms of our lack of knowledge of the definite state of that underlying reality. So I agree that it is clearly natural to look for such an explanation for quantum uncertainty (and people certainly have!). And Bohmian theory provides an explanation of this kind. So I guess it is perhaps more the odd features of the explanation that it gives, that I don't like. But one of these odd features is tightly connected to the non-observation of nonequilibrium phenomena---it is precisely the hiddenness, in general, of the Bohmian hidden variables, the fact that we apparently can't, or so far haven't, manipulate them directly, prepare or even observe any departures from the distribution over those variables that agrees with the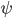 -function. I guess my comparison with statistical mechanics was also strongly influenced by having just reread Bohm's paper. I found it striking how much emphasis he laid on the interesting possibility of going beyond standard quantum theory to observe or manipulate the hidden variables directly. I still do think that nonequilibrium phenomena add to the compellingness of statistical mechanics. One could argue, I suppose, that fluctuations made it very plausible, but that the confirmation provided by statistical explanations of some nonequilibrium phenomena is important to our continued confidence in statistical mechanical explanations more generally.
-function. I guess my comparison with statistical mechanics was also strongly influenced by having just reread Bohm's paper. I found it striking how much emphasis he laid on the interesting possibility of going beyond standard quantum theory to observe or manipulate the hidden variables directly. I still do think that nonequilibrium phenomena add to the compellingness of statistical mechanics. One could argue, I suppose, that fluctuations made it very plausible, but that the confirmation provided by statistical explanations of some nonequilibrium phenomena is important to our continued confidence in statistical mechanical explanations more generally.
It might of course turn out, upon reading the various attempts, e.g. by Bohm and more recently by Dürr, Goldstein, and Zanghi in
http://arxiv.org/abs/quant-ph/0308039
to explain why the Bohmian position variable should be distributed as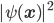 (and I guess also to explain why Bohmian position
(and I guess also to explain why Bohmian position 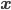 must be associated with Bohmian momentum
must be associated with Bohmian momentum 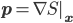 , where
, where 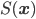 is the phase (arg
is the phase (arg 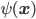 ) of the wavefunction at
) of the wavefunction at 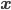 ), that I would also find an explanation of why it is so difficult to observe Bohmian systems out of "quantum equilibrium".
), that I would also find an explanation of why it is so difficult to observe Bohmian systems out of "quantum equilibrium".
Thanks again, Matt... food for more thought.
Howard